The following are the current texts and their table of contents for MS 090 Conceptual Mathematics, MS 095 PreAlgebra, and MS 098 Transition to Algebra. One might note that the contents for the MS 095 Carson text are the same as the proposed MS 090 outline contents. This issue of redundant coverage between the proposed MS 090 outline and the extant MS 095 outline is documented in a triple-column side-by-side synchronized study of the developmental math outlines.
Following the table are links to related texts at the Addison-Wesley site and links to topic areas at the Houghton Mifflin site.
Finally, there is material on a text that would span the current outlines of MS 095 and MS 098. The first nine chapters are the current MS 095 outline, the second nine chapters are the MS 098 outline. There was a time in the mid-1990s when we used a common text for two sequential developmental math courses, but then the text went out of print. This text would open up this possibility again.

Such a text could be used in the "option two" desynchronized option I outlined earlier today. In this second scenario, an elementary/secondary school text such as Saxon or Glencoe would continue to serve MS 090, which would intake only the weakest of the weak students. The "mode" for placement would be MS 095 and the Bittinger/Ellenbogen text. MS 090 would be limited to a max of 20 students per section, while MS 095 and MS 098 would run at a 30 student per section limit. Students coming out of MS 090 would probably be primarily placed into MS 095, but the top students might be moved into MS 098 directly.
Under "Option two" the MS 090 student learning outcomes would have to be adjusted in some way to make it clear that the level of the course was below that of MS 095.
For reference the original 1997 MS 090 outline remains on line, as does the extant MS 098 outline and the newly approved MS 100 outline.
| MS 090 | MS 095 | MS098 |
|---|---|---|
 Saxon does not provide an on line table of contents that I could find to cut and paste into this table Saxon Math 8/7 with Pre-Algebra Saxon Math 8/7 builds on arithmetic calculation, measurements, basic geometry and other foundational concepts and skills. Saxon Math 8/7 also includes extensive prealgebra exercises, preparing the student for upper-level mathematics. |
 Note: Each ends with a Summary, Review Exercises, and a Chapter Test. Starting with Chapter 2, each chapter also ends with a Cumulative Review. Chapter 1. Whole Numbers Introduction to Numbers, Notation, and Rounding Adding, Subtracting, and Solving Equations with Whole Numbers Multiplying Whole Numbers and Exponents Dividing, Square Roots, and Solving Equations with Whole Numbers Order of Operations Variables, Formulas, and Solving Equations More with Problem Solving and Applications Chapter 2. Integers Introduction to Integers Adding Integers Subtracting Integers and Solving Equations Multiplying, Dividing, and Solving Equations with Integers Order of Operations Applications and Problem Solving Chapter 3. Expressions and Polynomials Evaluating Expressions Introduction to Polynomials Simplifying Polynomials Adding and Subtracting Polynomials Multiplying Polynomials Prime Numbers and GCF Introduction to Factoring Applications and Problem Solving Chapter 4. Equations Equations and Their Solutions The Addition/Subtraction Principle of Equality The Multiplication/Division Principle of Equality Translating Word Sentences to Equations Applications and Problem Solving Chapter 5. Fractions and Rational Expressions Fractions, Mixed Numbers, and Rational Expressions Simplifying Fractions and Rational Expressions Multiplying Fractions, Mixed Numbers, and Rational Expressions Dividing Fractions, Mixed Numbers, and Rational Expressions Least Common Multiple Adding and Subtracting Fractions, Mixed Numbers, and Rational Expressions. Order of Operations and Evaluating and Simplifying Expressions Solving Equations Chapter 6. Decimals Decimals and Rational Numbers Adding and Subtracting with Decimals and Solving Equations Multiplying Decimal Numbers; Exponents with Decimal Bases Dividing and Roots with Decimals Order of Operations and Applications in Geometry Solving Equations and Problem Solving Chapter 7. Ratios, Proportions, and Measurement Ratios, Probability, and Rates Proportions American Measurement Metric Measurement Converting Between the American and Metric Systems Applications and Problem Solving Chapter 8. Percents Introduction to Percent Translating Percent Sentences Word-for-Word Translating Percent Sentences to Proportions Solving Percent Problems (Portions) Solving Problems Involving Percent of Increase or Decrease Solving Problems Involving Interest Chapter 9. Statistics and Graphs Mean, Median, and Mode Tables, Charts, and Graphs The Rectangular Coordinate System Graphing Linear Equations Applications with Graphing Appendix: Answers to Odd-Numbered Exercises, All Review Exercises, and All Practice Test Problems Glossary Index |
 ContentsNote: Each chapter begins with a Prep Test and concludes with a Summary, Review Exercises, and Test. Chapters 2-6 are followed by Cumulative Review Exercises.1. Prealgebra Review Section 1.1 Introduction to Integers Section 1.2 Addition and Subtraction of Integers Section 1.3 Multiplication and Division of Integers Section 1.4 Exponents and the Order of Operations Agreement Section 1.5 Factoring Numbers and Prime Factorization Section 1.6 Addition and Subtraction of Rational Numbers Section 1.7 Multiplication and Division of Rational Numbers Section 1.8 Concepts from Geometry Focus on Problem Solving: Inductive Reasoning Projects and Group Activities: The +/- Key on a Calculator 2. Variable Expressions Section 2.1 Evaluating Variable Expressions Section 2.2 Simplifying Variable Expressions Section 2.3 Translating Verbal Expressions into Variable Expressions Focus on Problem Solving: From Concrete to Abstract Projects and Group Activities: Prime and Composite Numbers 3. Solving Equations Section 3.1 Introduction to Equations Section 3.2 General Equations--Part I Section 3.3 General Equations--Part II Section 3.4 Translating Sentences into Equations Section 3.5 Geometry Problems Section 3.6 Mixture and Uniform Motion Problems Focus on Problem Solving: Trial-and-Error Approach to Problem Solving Projects and Group Activities: Nielsen Ratings 4. Polynomials Section 4.1 Addition and Subtraction of Polynomials Section 4.2 Multiplication of Monomials Section 4.3 Multiplication of Polynomials Section 4.4 Integer Exponents and Scientific Notation Section 4.5 Division of Polynomials Focus on Problem Solving: Dimensional Analysis Projects and Group Activities: Diagramming the Square of a Binomial 5. Factoring Section 5.1 Common Factors Section 5.2 Factoring Polynomials of the Form x2 + bx + c Section 5.3 Factoring Polynomials of the Form ax2 + bx + c Section 5.4 Special Factoring Section 5.5 Solving Equations Focus on Problem Solving: Making a Table Projects and Group Activities: Evaluating Polynomials Using a Graphing Calculator 6. Rational Expressions Section 6.1 Multiplication and Division of Rational Expressions Section 6.2 Expressing Fractions in Terms of the Least Common Multiple (LCM) Section 6.3 Addition and Subtraction of Rational Expressions Section 6.4 Complex Fractions Section 6.5 Solving Equations Containing Fractions Section 6.6 Ratio and Proportion Section 6.7 Literal Equations Section 6.8 Application Problems Focus on Problem Solving: Negations and If. . .then Sentences Projects and Group Activities: Intensity of Illumination 7. Linear Equations in Two Variables Section 7.1 The Rectangular Coordinate System Section 7.2 Linear Equations in Two Variables Section 7.3 Intercepts and Slopes of Straight Lines Section 7.4 Equations of Straight Lines Focus on Problem Solving: Counterexamples Projects and Group Activities: Graphing Linear Equations with a Graphing Utility 8. Systems of Linear Equations Section 8.1 Solving Systems of Linear Equations by Graphing Section 8.2 Solving Systems of Linear Equations by the Substitution Method Section 8.3 Solving Systems of Equations by the Addition Method Section 8.4 Application Problems in Two Variables Focus on Problem Solving: Using a Table and Searching for a Pattern Projects and Group Activities: Finding a Pattern 9. Inequalities Section 9.1 Sets Section 9.2 The Addition and Multiplication Properties of Inequalities Section 9.3 General Inequalities Section 9.4 Graphing Linear Inequalities Focus on Problem Solving: Graphing Data Projects and Group Activities: Mean and Standard Deviation 10. Radical Expressions Section 10.1 Introduction to Radical Expressions Section 10.2 Addition and Subtraction of Radical Expressions Section 10.3 Multiplication and Division of Radical Expressions Section 10.4 Solving Equations Containing Radical Expressions Focus on Problem Solving: Deductive Reasoning Projects and Group Activities: Distance to the Horizon 11. Quadratic Equations Section 11.1 Solving Quadratic Equations by Factoring or by Taking Square Roots Section 11.2 Solving Quadratic Equations by Completing the Square Section 11.3 Solving Quadratic Equations By Using the Quadratic Formula Section 11.4 Graphing Quadratic Equations in Two Variables Section 11.5 Application Problems Focus on Problem Solving: Algebraic Manipulation and Graphing Techniques Projects and Group Activities: Graphical Solutions of Quadratic Equations |
Note: Saxon Publishers must have recently been purchased by Harcourt Achieve as the Saxonpublishers.com web site is now redirected to the Harcourt Achieve web site.



Prealgebra 2e by Carson © 2006
ISBN:
0-321-23736-6
Prealgebra 3e by Lial/Hestwood © 2006 ISBN:
0-321-26662-5
Integrated Arithmetic and Basic Algebra, 3e by Jordan/Palow © 2005 ISBN:
0-321-13226-2
Basic Mathematics
Prealgebra
Beginning Algebra
Intermediate Algebra
Algebra for College Students
College Algebra
College Algebra and Trigonometry
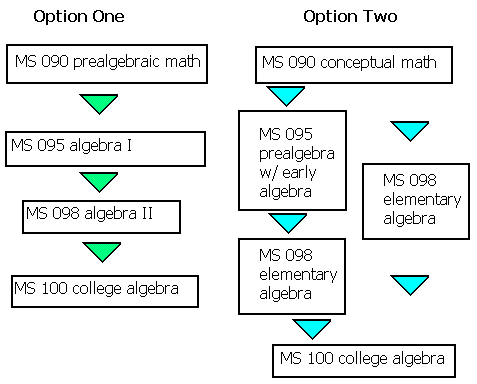
In option one MS 090 is PreAlgebra, in option two MS 090 is a small section size mathematics course that ranges from arithmetic to algebra in a series of spirals from concrete models to abstract algebraic systems. Under option two MS 090 is "desequenced," students are then placed into the appropriate course in the "regular developmental curriculum."
| Option One | Option Two |
|---|---|
| MS 095 Elementary Algebra I MS 098 Elementary Algebra II |
MS 095 PreAlgebra MS 098 Transition to Algebra |

Elementary and Intermediate Algebra |
Prealgebra and Introductory Algebra Marvin L. Bittinger, Indiana University Purdue University Indianapolis |
|
Description Elementary and Intermediate Algebra is a book for the student. The authors' goal is to help build students' confidence, their understanding and appreciation of math, and their basic skills by presenting an extremely user-friendly text that models a framework in which students can succeed. Unfortunately, students who place into developmental math courses often struggle with math anxiety due to bad experiences in past math courses. Developmental students often have never developed nor applied a study system in mathematics. To address these needs, the authors have framed three goals for Elementary and Intermediate Algebra: 1) reduce math anxiety, 2) teach for understanding, and 3) foster critical thinking and enthusiasm. The authors' writing style is extremely student friendly. They talk to students in their own language and walk them through the concepts, explaining not only how to do the math, but also why it works and where it comes from, rather than using the "monkey-see, monkey-do" approach that some books take. |
Description Prealgebra and Introductory Algebra, 1e is a new offering from a trusted author team and publisher. This text covers all of the core material that is typically found in both a Prealgebra and an Introductory Algebra course, all in one textbook. And, as you have come to expect when you see the Bittinger name, Prealgebra and Introductory Algebra, 1e offers you and your students the Bittinger hallmark five-step problem-solving process, a clear easy-to-read writing style, real-data applications, and exceptional design and artwork to make learning interesting and fun. |
|
Table of Contents 1. Foundations of Algebra. |
Table of Contents (All chapters begin with a Pretest and end with a Summary and Review, Chapter Test, and, with the exception of Chapter 1, a Cumulative Review). |